
Sooner or later you will want to leave the local area and go somewhere in your glider. The first time you realize that you are out of gliding range of the airport will feel a lot like that first solo. Most likely you will plan that first cross-country flight as thoroughly as any you will ever attempt. The starting place for that planning probably will be a Sectional Chart, because, at a scale of 1:500,000, it provides a good trade off between detailed information and adequate coverage. Area charts, scale 1:250,000, provide more detail for flights near major airports and World Aeronautical Charts (WACs), scale 1:1,000,000, provide more coverage.
 | An essential element of navigation is an understanding of latitude and longitude as a means of uniquely identifying any point on the earth. Parallels of latitude start at zero at the equator and increase both north and south to 90o at the poles. Meridians of longitude start at zero at the "Prime Meridian" through Greenwich, England and increase east and west to 180o. |
The earth is also divided into 24 time zones, which correspond roughly to 15o longitude segments. Universal Coordinated Time (UTC) is used in aviation to minimize confusion created by different time zones. UTC is based on the time at 0o longitude and is sometimes called "Zulu" time, indicated by the letter Z following the time. To convert from local time to UTC use the following factors:
| TIME ZONE | STANDARD TIME ADD HOURS | DAYLIGHT SAVING TIME ADD HOURS |
|---|---|---|
| EASTERN | 5 | 4 |
| CENTRAL | 6 | 5 |
| MOUNTAIN | 7 | 6 |
| PACIFIC | 8 | 7 |
There is no way to display a spherical surface such as the earth's on a flat chart without losing a little accuracy, but aeronautical charts come close. If you examine this kind of chart carefully you will find that the meridians are straight lines that converge toward the poles (they actually do meet at the poles on the sphere). Parallels of latitude curve slightly.
 Gliders rarely fly straight courses for very far because thermals rarely line up with where you want to go, but if you do want to measure the direction of a course, measure at a meridian near the midpoint, as shown here, to get the most accurate measurement. The meridians are pointing toward true north so the direction you get by measuring this way gives you the true course. You probably would rather have magnetic course so you must add westerly or subtract easterly variation as shown on the nearest magenta colored isogonic line. The memory aid, "East is Least and West is Best", is supposed to help you remember to subtract east and add west to convert from true to magnetic direction. If you should want to convert from magnetic to true, maybe because of a test question, then remember to add east and subtract west.
Gliders rarely fly straight courses for very far because thermals rarely line up with where you want to go, but if you do want to measure the direction of a course, measure at a meridian near the midpoint, as shown here, to get the most accurate measurement. The meridians are pointing toward true north so the direction you get by measuring this way gives you the true course. You probably would rather have magnetic course so you must add westerly or subtract easterly variation as shown on the nearest magenta colored isogonic line. The memory aid, "East is Least and West is Best", is supposed to help you remember to subtract east and add west to convert from true to magnetic direction. If you should want to convert from magnetic to true, maybe because of a test question, then remember to add east and subtract west.
A plotter similar to the one in our illustration is an essential tool for flight planning. It is used to measure distances as well as angles. When doing so it is a good idea to compare the scale on the plotter with the scale on the chart and make adjustments as needed. That is especially true if you obtained the chart by printing it from your computer.
Aeronautical navigation is divided into three categories. Radio navigation, except for GPS, is not often used in glider flying. It is discussed in the section on "Systems" to provide enough information to answer most of the knowledge test questions. Pilotage, navigation by reference to objects on the ground is probably the most popular for glider pilots. It relies heavily on the Sectional Chart, which will be discussed in more detail later. The third type of navigation is dead reckoning. This involves preplanning the flight to include the effect of wind on the selected course. The result is a magnetic heading that will result in the aircraft following the desired course although it may be pointed in a different direction. Classic dead reckoning doesn't apply very well to glider cross-country flights because of the need to alter course frequently to reach sources of lift. However, it is important for all pilots to understand the concept of a wind triangle.
Because of our lifetime of experience moving about on the ground we tend to judge our motion relative to the ground even when we are in the air. Motion relative to the ground is important, of course, because we usually want to go from one point on the ground to another. But we also need to be aware of our motion relative to the air we fly in because that is all the glider understands. Failure to do so may be the reason for many tragic landing accidents, where proximity to the ground makes it hard to distinguish between motion relative to the air and motion relative to the ground.
 The wind triangle is another application of vectors. When we discussed vectors as applied to aerodynamics we noted that they provided a way to see the effect of two forces that acted in different directions. In the wind triangle we will see how two motions, motion of the glider through the air and motion of the air over the ground (wind), combine to give us motion of the glider over the ground. In this illustration we see how a glider moving east through air that is moving southwest (a northeast wind) moves over the ground in a southeasterly direction.
The wind triangle is another application of vectors. When we discussed vectors as applied to aerodynamics we noted that they provided a way to see the effect of two forces that acted in different directions. In the wind triangle we will see how two motions, motion of the glider through the air and motion of the air over the ground (wind), combine to give us motion of the glider over the ground. In this illustration we see how a glider moving east through air that is moving southwest (a northeast wind) moves over the ground in a southeasterly direction.
 If the pilot really wants to go east it is necessary to compensate for the wind by crabbing into it. The upper blue line represents the direction and speed of the glider through the air. The lower blue line represents the wind direction and speed. The red line is their resultant, the direction and speed of the glider over the ground. Note, as with all vector problems, we construct a parallelogram where two sides represent the two forces or motions we want to combine. The diagonal of this parallelogram, the red line in our illustration, represents the combined effect of them.
If the pilot really wants to go east it is necessary to compensate for the wind by crabbing into it. The upper blue line represents the direction and speed of the glider through the air. The lower blue line represents the wind direction and speed. The red line is their resultant, the direction and speed of the glider over the ground. Note, as with all vector problems, we construct a parallelogram where two sides represent the two forces or motions we want to combine. The diagonal of this parallelogram, the red line in our illustration, represents the combined effect of them.
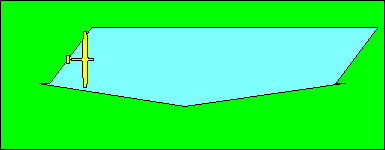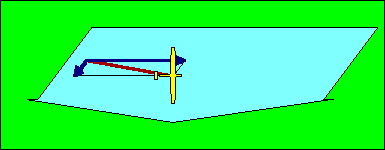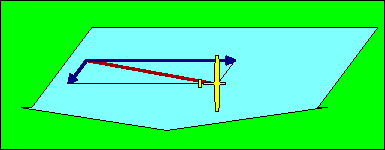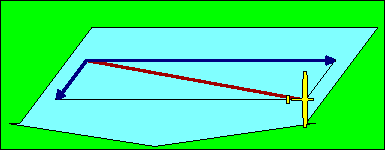
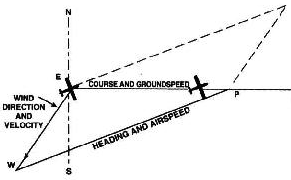 This illustration from the "Pilot's Handbook of Aeronautical Knowledge" is how the wind triangle looks in a classic dead reckoning problem. There are six elements in these problems: the direction you want to go (course), the speed along the course (groundspeed), the wind direction, the wind speed, the direction the aircraft must be pointed (heading), and its speed (airspeed). If you know any four of these, you can find the other two with the wind triangle. Usually you know the course, wind direction, wind speed, and airspeed. You want to find heading and groundspeed. As illustrated here, you lay out the course line and the wind direction and speed. From the end of the wind vector construct an arc with radius equal to the airspeed. From where the arc intersects the course line to the origin represents the groundspeed and a line from that point to the end of the wind vector represents the heading. The dashed lines complete the parallelogram but are really not needed to work the problem.
This illustration from the "Pilot's Handbook of Aeronautical Knowledge" is how the wind triangle looks in a classic dead reckoning problem. There are six elements in these problems: the direction you want to go (course), the speed along the course (groundspeed), the wind direction, the wind speed, the direction the aircraft must be pointed (heading), and its speed (airspeed). If you know any four of these, you can find the other two with the wind triangle. Usually you know the course, wind direction, wind speed, and airspeed. You want to find heading and groundspeed. As illustrated here, you lay out the course line and the wind direction and speed. From the end of the wind vector construct an arc with radius equal to the airspeed. From where the arc intersects the course line to the origin represents the groundspeed and a line from that point to the end of the wind vector represents the heading. The dashed lines complete the parallelogram but are really not needed to work the problem.
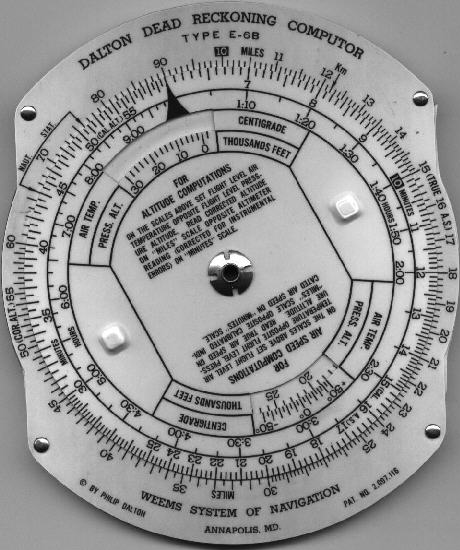 The E-6B Dead Reckoning Computer is a tool of the slide rule era for working wind triangle problems easily. It is unlikely that you will need to use that capability either for planning glider flights or taking knowledge tests. The calculator side of the E-6B is really a circular slide rule and is useful for glider calculations.
The E-6B Dead Reckoning Computer is a tool of the slide rule era for working wind triangle problems easily. It is unlikely that you will need to use that capability either for planning glider flights or taking knowledge tests. The calculator side of the E-6B is really a circular slide rule and is useful for glider calculations.
Many problems are concerned with time-speed-distance relationships. These are easily solved by placing the speed index (that black pointer on the inner scale) opposite the speed on the outer scale. Then distance and time can be read opposite each other on the outer and inner scales, respectively. In this illustration, if your speed is 90 KTS (or MPH) then it takes 90 minutes or 1:30 hours to go 135 NM (or SM). Conversely, you can go 135 NM in an hour and a half at 90 KTS.
A good application of this computer to glider flying is to relate nautical miles traveled to feet of altitude lost at a given glide ratio. Set the speed index under the glide ratio and read distance traveled on the outer scale opposite feet altitude lost on the inner scale. Since we can't adjust the computer in the illustration, pretend we have a fantastic glide ratio of 90:1 and can travel 120 NM with an altitude loss of 8,000 feet.
When using a "slide rule" type computer it is necessary to be careful about interpreting the value of a number on the scale. The number 90 in our first example represented 90 KTS, but the number 13.5 actually meant 135 NM. The printed values can represent the actual number or the value multiplied or divided by any multiple of 10. In the days when slide rules hung from every engineer's belt they got very good at adding and subtracting powers of 10 to arrive at the correct interpretation. Today, it may be better to just use an electronic calculator.
Pilotage involves relating what you see out the window with what you see on a chart. Pilots should study the legend on the Sectional Chart until they are familiar with the meaning of all the symbols on the chart. Airports are good checkpoints but it takes some practice to be able to recognize them from a distance. Mountain peaks look a lot alike and are hard to distinguish unless they are isolated. Lakes and rivers may change appearance significantly at different times of the year. Sectional Charts show the shapes of large towns and cities but small ones are better identified by highway and railroad patterns around them.

| The elevation of the terrain is shown by contour lines and color-coding. The interval between color changes is shown on the front of the chart. Actual contour lines are labeled with their elevation, as are spot elevations of peaks. The maximum elevation figure (MEF) represents the highest known feature in each quadrangle bounded by lines of latitude and longitude. | 
|
 Obstructions such as towers are shown by symbols that resemble towers and include tiny lightning bolts if they have high-intensity lights. The elevation shown is the top of the obstruction above MSL. Another number in parenthesis shows the height AGL. Because supporting wires may extend some distance from
obstructions, they should be avoided by 2,000 feet horizontally.
Obstructions such as towers are shown by symbols that resemble towers and include tiny lightning bolts if they have high-intensity lights. The elevation shown is the top of the obstruction above MSL. Another number in parenthesis shows the height AGL. Because supporting wires may extend some distance from
obstructions, they should be avoided by 2,000 feet horizontally.
If you get lost and the only avionics equipment you have is a VHF radio, you can get help from a Flight Service Station (FSS) offering Direction Finding Service (DF). This service allows the FSS personnel to determine your direction from two of their ground stations. Your location is where the two directions intersect. All you need to do is call them, ask for a DF fix, and follow instructions.
If you plan to go farther than you can glide from release from tow, you need to find some air moving upward. The most popular cross-country lift source is thermal lift. Except for a few reports of thermals caused by large fires or industrial facilities, most thermals depend on solar heating. Cumulus clouds are the classic indicators of thermals but strong thermals can exist in clear air if the humidity levels do not support cloud formation. It is also true that the cloud may last after the thermal that created it is no longer useful for glider pilots. A concave underside of the cloud may indicate that the thermal is still active and where it is strongest.
Other indicators of thermals are found at the surface. Dark areas are better than light ones; dry areas are better than wet ones; and clear areas are better than those with vegetation. Greater solar heating will occur when the sunlight strikes a surface directly rather than at an angle, so thermals are more likely on the eastern side of a hill in the morning and the western side in the afternoon. Since air must enter the base of the thermal to sustain it, converging dust or smoke is a good indicator. In drier climates it is common to find a column of rising dust indicating a thermal. Usually called dust devils, these can be a good source of lift but a hazard near the surface. "Aviation Weather", quoting an Egyptian flight instructor, recommends approaching a dust devil opposite its direction of rotation, 500 feet or more AGL, and staying out of the eye of the vortex.
Soaring birds often are good indicators of thermals. However, bubble thermals, the result of slow or intermittent heating, may mean that there is no thermal for the glider pilot who moves in below the birds. Bubble thermals are an excellent explanation for passengers when the glider pilot just fell out of a thermal. Following sea gulls out to sea is not recommended, but if you do go farther than you can glide back and have a paying passenger on board, be aware that regulations require you to carry flotation gear for each occupant.
Thermals tilt with the wind so their location at the glider's altitude likely will not be directly over or under the indicator that attracted the pilot to the thermal initially.
Thermals are more likely to be found in summer than winter, but heat alone is no guarantee of good soaring conditions. The stability of the air is the most important factor so after a prolonged period of heat, the temperatures aloft may have risen so much that thermal indexes are positive and soaring impossible. The best solution for this problem is for a cold front to pass through and replace the warm air with colder air and restore unstable lapse rates.
A cloud street or thermal street is a line of thermals that permits cross-country flight with little or no need to stop and circle. Slowing to the speed-to-fly for the thermal climb rate, or at least slowing to minimum sink speed, is appropriate while passing through the thermal. If you decide to stop and circle, the speed to fly is a trade-off involving several factors. The diameter of the thermal and the distribution of lift across it determine the optimum circling radius for the glider. Generally the smaller the turn radius the stronger the lift, but the glider performance decreases. One might think (and the author of the FAA knowledge tests does) that the correct speed would be the minimum sink speed for the bank angle. Remember that minimum sink speed, as all performance speeds do, increases in proportion to the square root of the load factor, which increases with bank angle. However, turn radius is dependent on both the tangent of bank angle and the speed squared. Thus flying a little slower may permit a smaller turn radius for the same bank angle. The glider's performance curve is relatively flat for a few knots either side of the minimum sink speed so flying a little slower than minimum sink does not incur a great sink penalty. Of course a safe margin above stall speed must be maintained or any benefit from flying a little slower will be erased quickly. That is why we practice turning stalls so that lowering the nose and picking up the wing with rudder become automatic at the first sign of stall.
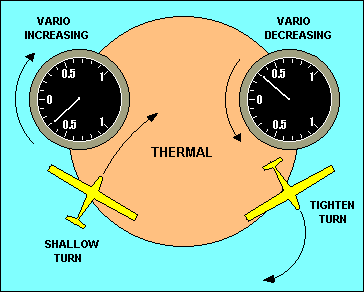 Safe thermaling requires that pilots entering a thermal with another glider turn in the same direction and position themselves so they can maintain visual contact with the other glider. Each pilot develops a unique technique for thermaling, but all are based on developing a mental picture of where the rising air is and maneuvering the glider into that air. The variometer is the most useful instrument in developing this picture but pilots must understand its lag in showing actual vertical speed.
Safe thermaling requires that pilots entering a thermal with another glider turn in the same direction and position themselves so they can maintain visual contact with the other glider. Each pilot develops a unique technique for thermaling, but all are based on developing a mental picture of where the rising air is and maneuvering the glider into that air. The variometer is the most useful instrument in developing this picture but pilots must understand its lag in showing actual vertical speed.
A good technique for beginners is illustrated here. The glider on the right has just flown out of the thermal into sinking air but its variometer still shows lift due to lag. The pilot should increase bank (think "turn away from sink") as soon as the variometer starts down, instead of waiting for it to show sink. Similarly, when the glider reaches the point on the left the pilot should decrease bank (think "do not turn away from lift") as soon as the variometer starts back up. By anticipating what the variometer is going to show in a few seconds, the pilot is compensating for its lag.
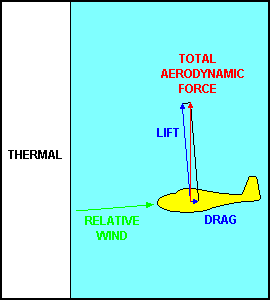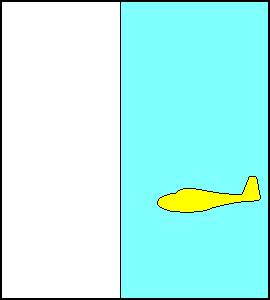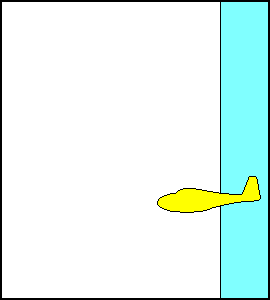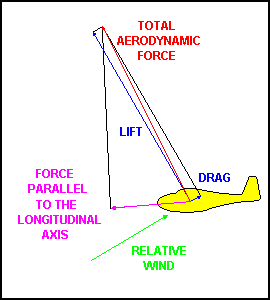 Sometimes the entry to a thermal is indicated by an increase in airspeed.
In this illustration the forces on the glider flying in "blue" air are balanced.
It is descending at a constant speed and the red resultant of lift and drag just equals weight.
Its flight path is exactly opposite to the relative wind and its wing is operating at a modest angle of attack.
When it suddenly enters the thermal, all that changes rather abruptly.
The vertical motion of the air in the thermal combined with the forward speed of the glider changes the direction of the relative wind and results in a significant increase in angle of attack.
That produces a large increase in both lift and drag, but more importantly, it changes the direction those forces act.
Remember that lift is perpendicular to the relative wind and drag is parallel to it.
Their resultant, the total aerodynamic force on the glider, is now tipped forward relative to the glider's longitudinal axis.
It is the component of this force, parallel to the longitudinal axis, that accelerates the glider.
Of course there is also a large component acting perpendicular to the longitudinal axis and it is this force that produces that wonderful feeling in the seat of the pilots pants that says, "This is a good one."
Sometimes the entry to a thermal is indicated by an increase in airspeed.
In this illustration the forces on the glider flying in "blue" air are balanced.
It is descending at a constant speed and the red resultant of lift and drag just equals weight.
Its flight path is exactly opposite to the relative wind and its wing is operating at a modest angle of attack.
When it suddenly enters the thermal, all that changes rather abruptly.
The vertical motion of the air in the thermal combined with the forward speed of the glider changes the direction of the relative wind and results in a significant increase in angle of attack.
That produces a large increase in both lift and drag, but more importantly, it changes the direction those forces act.
Remember that lift is perpendicular to the relative wind and drag is parallel to it.
Their resultant, the total aerodynamic force on the glider, is now tipped forward relative to the glider's longitudinal axis.
It is the component of this force, parallel to the longitudinal axis, that accelerates the glider.
Of course there is also a large component acting perpendicular to the longitudinal axis and it is this force that produces that wonderful feeling in the seat of the pilots pants that says, "This is a good one."
In the section on aerodynamics we defined "speed-to-fly" as the speed that will produce the flattest glide over the ground. If you only want to know enough about performance airspeeds to pass the FAA knowledge and practical tests you probably could stop here. The FAA wants you to understand the concepts well enough so you don't do something dumb like trying to stretch a glide by pulling back on the stick when you should be pushing forward.
|
|
Although the Speeds to Fly that have been
discussed previously are the ones that will let you glide to that safe
landing spot if any will, they are too slow to win contests and too
slow for most cross-country flights. In these cases we are more
interested in covering the ground in the shortest time rather than
obtaining the flattest glide. That introduces a new definition of
Speed to Fly. Credit for developing this concept of Speed to Fly is usually given to Paul MacCready, and the speed ring discussed earlier is usually called a MacCready speed ring. Cross-country flights utilizing thermals consist of alternately climbing in one thermal and gliding to the next. MacCready noted that the Speed to Fly between thermals should be based on the strength of the thermals. If the thermals are strong you can fly faster than the speed that produces the flattest glide. You will lose more altitude, but you make up for it by climbing faster in the next thermal. Thus you reach the top of the next thermal sooner than you would have at the lower speed. |
|
| We can analyze Speed to Fly in this case by using the same technique we used for flattest glide in sinking air, except that we offset the polar curve origin by the rate of climb we expect in the next thermal instead of air mass sink rate. To illustrate the similarity we assume a climb rate of 3 kts and see that the graph is identical to the one with 3 kts sink except for the labels. It shows that the Speed to Fly between thermals in still air is 62 kts if you expect to climb at 3 kts in the next thermal. An added benefit is that the average cross-country speed, including the time spent circling in thermals, can be estimated by the point on the airspeed scale where the tangent line crosses the scale. |
Now we see why the speed ring can be rotated about the variometer. If we expect our climb rate to be 3 kts, we set the arrow on the ring opposite where the vario indicates 3 kts climb. Note that the Speeds to Fly also move so that a higher speed is indicated for all sink rates. In this example the Speed to Fly in still air is 62 kts, just as the graph predicted. The sink rate in still air at this speed is 3.2 kts.
|
| Some pilots might question using a climb rate in the next thermal, one that has not yet been attained, to set the speed ring. They should try to remember the last time they made a decision with complete information. In this case one could base the estimate on the climb rate in the last thermal, modified by an assessment of conditions ahead and whether they appear to be improving or deteriorating. Once set, the value selected is still subject to change. A good way to think of the MacCready setting is that it represents the minimum climb rate that the pilot is willing to stop for. As altitude decreases and the prospects of landing out increase, the prudent pilot will accept weaker thermals, and the MacCready ring should be set accordingly. A really desperate pilot should set the ring to zero to maximize the area to be searched for a thermal. |
 Before leaving the subject of cross-country thermal soaring we need to discuss the effect of wind. Increasing speed with a headwind improves glide performance relative to the ground. However, since thermals are part of the air mass, they are affected by wind the same as the glider flying in it. Thus when flying from thermal to thermal the pilot can ignore the effect of wind. It does become important when flying to a point on the ground. The most common example of this is the "final glide" from the last thermal to the landing spot.
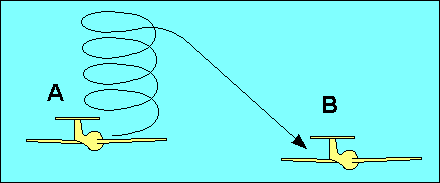
Imagine the glider in this illustration at point `A' climbing in the last thermal before gliding to a landing at point `B'. The pilot could leave the thermal with just enough altitude to reach `B', but it might be better to continue climbing and fly faster during the glide. The optimum speed-to-fly from `A' to `B' depends on the glide performance of the glider, the strength of the thermal and the wind. The altitude to reach before leaving the thermal depends on these plus the distance to go and the target altitude at `B'. Simple "Final Glide Calculators", which look like a circular slide rule, are available for most gliders to aid the pilot in solving this problem.
Before leaving the subject of cross-country thermal soaring we need to discuss the effect of wind. Increasing speed with a headwind improves glide performance relative to the ground. However, since thermals are part of the air mass, they are affected by wind the same as the glider flying in it. Thus when flying from thermal to thermal the pilot can ignore the effect of wind. It does become important when flying to a point on the ground. The most common example of this is the "final glide" from the last thermal to the landing spot.
Imagine the glider in this illustration at point `A' climbing in the last thermal before gliding to a landing at point `B'. The pilot could leave the thermal with just enough altitude to reach `B', but it might be better to continue climbing and fly faster during the glide. The optimum speed-to-fly from `A' to `B' depends on the glide performance of the glider, the strength of the thermal and the wind. The altitude to reach before leaving the thermal depends on these plus the distance to go and the target altitude at `B'. Simple "Final Glide Calculators", which look like a circular slide rule, are available for most gliders to aid the pilot in solving this problem.
The cross-country glider pilot should be prepared for landing at sites other than airports. A good rule is to have an area selected at 2,000 feet agl and the specific field at 1,500 feet agl. Obviously the smaller the rocks and the shorter the vegetation the better, and don't be surprised if they grow significantly as you approach for landing. Landing uphill is generally better than downhill even if it means landing downwind. After landing out the pilot likely will need to begin disassembly of the glider in preparation for its retrieval. At one time this was considered a preventive maintenance item. In 1987 glider assembly and disassembly were redefined as pilot operations and appropriate training was mandated.
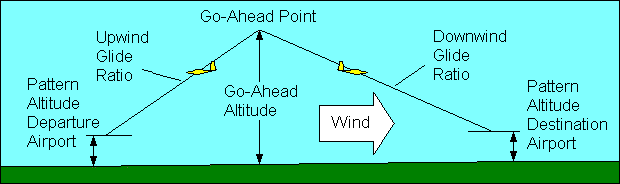
The probability of landing out can be reduced by constructing a flight profile to determine "go-ahead points". A go-ahead point is a location and altitude from which you can "go-ahead" to the next landing site and no longer consider returning to the previous one. It is determined by discounting the glide ratio relative to the ground (usually 50%) and calculating the altitude necessary to reach pattern altitude at each landing site from varying distances. The effect of wind on glide ratio is easily determined by multiplying the no-wind glide ratio by the ratio of ground speed to airspeed. Text books illustrate go-ahead points with a profile view of the flight drawn to scale, but its actual application is better implemented as concentric circles drawn on the navigation chart about the landing sites and labeled with the required altitudes.
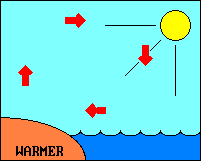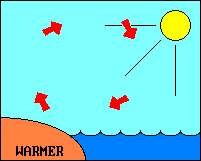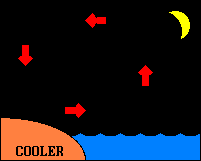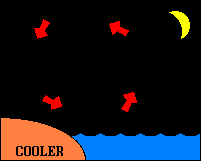 Land absorbs and radiates heat faster than water, causing temperature and pressure differences.
The circulation of air near a large body of water, rising over warmer land and sinking over cooler water during the day and reversing at night, provides "lift" identified by "land and sea breezes".
"The sea breeze usually begins during early forenoon, reaches a maximum during the afternoon, and subsides around dusk after the land has cooled. The leading edge of the cool sea breeze forces warmer air inland to rise."
(2)
Land absorbs and radiates heat faster than water, causing temperature and pressure differences.
The circulation of air near a large body of water, rising over warmer land and sinking over cooler water during the day and reversing at night, provides "lift" identified by "land and sea breezes".
"The sea breeze usually begins during early forenoon, reaches a maximum during the afternoon, and subsides around dusk after the land has cooled. The leading edge of the cool sea breeze forces warmer air inland to rise."
(2)
The cooling of the ground at night due to radiation also results in "mountain breezes", a relatively thin layer of cold air flowing down the mountain like a river of air. The reverse flow after daytime heating is called a "valley breeze" and the air flowing up the mountain can sometimes be utilized as a source of lift. For others who have trouble remembering which name goes with which breeze, the rule is that wind is always described by the direction from which it is coming; a west wind comes from the west, a sea breeze comes from the sea and a mountain breeze comes from the mountain.
 While all atmospheric movement can be traced ultimately to heating from the sun, thermal activity is a local phenomenon while the prevailing westerlies represent world scale air movement. When the local air movement is primarily horizontal (wind), it may be possible to utilize orographic lift for glider flying. Commonly called ridge lift, this deflection of the air upward over a hill or ridge can provide useful lift well above the height of the ridge. A general rule requires a wind speed of 15 knots or more at an angle to the ridge within 30 to 40 degrees of perpendicular for good ridge soaring.
Ridge soaring rules require all turns to be made into the wind. Passing should be between the other glider and the ridge, and flying directly over or under another glider is forbidden. If a ridge must be approached from the downwind side it should be with excess altitude and it should be done at an angle to facilitate turning away if necessary.
While all atmospheric movement can be traced ultimately to heating from the sun, thermal activity is a local phenomenon while the prevailing westerlies represent world scale air movement. When the local air movement is primarily horizontal (wind), it may be possible to utilize orographic lift for glider flying. Commonly called ridge lift, this deflection of the air upward over a hill or ridge can provide useful lift well above the height of the ridge. A general rule requires a wind speed of 15 knots or more at an angle to the ridge within 30 to 40 degrees of perpendicular for good ridge soaring.
Ridge soaring rules require all turns to be made into the wind. Passing should be between the other glider and the ridge, and flying directly over or under another glider is forbidden. If a ridge must be approached from the downwind side it should be with excess altitude and it should be done at an angle to facilitate turning away if necessary.
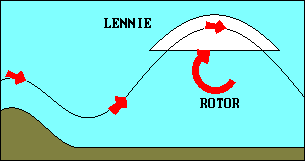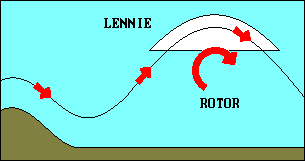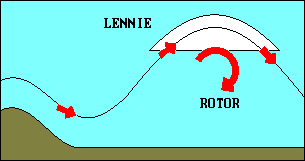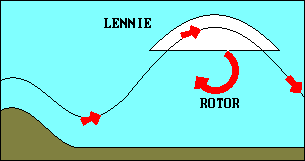 The area immediately downwind from a ridge is usually dangerously turbulent sinking air. However, under certain conditions, somewhat farther downwind the air may start to rise to form a mountain wave.
"A strong mountain wave requires: 1. Marked stability in the airstream disturbed by the mountains. 2. Wind speed at the level of the summit should exceed a minimum that varies from 15 to 25 knots. 3. Wind direction should be within 30 degrees normal to the range."
(2)
Actually several waves may form with some many miles downwind from the mountain causing them. The rising portion of these standing waves (the wave itself is stationary although the air is moving through it at high speed) often provides a spectacular source of lift. Air rising in a wave obeys the same physical laws as that in a thermal, cooling at 3 C per 1,000 feet. Thus the wave may be marked by a lens shaped cloud, standing lenticular altocumulus, or "lennie", which forms on the upwind side by condensation of moisture as the rising air reaches its dew point and dissipates on the downwind side as the sinking air warms. Directly beneath the wave will be an area of extreme turbulence called the rotor, which may or may not be marked by a tumbling cloud.
The area immediately downwind from a ridge is usually dangerously turbulent sinking air. However, under certain conditions, somewhat farther downwind the air may start to rise to form a mountain wave.
"A strong mountain wave requires: 1. Marked stability in the airstream disturbed by the mountains. 2. Wind speed at the level of the summit should exceed a minimum that varies from 15 to 25 knots. 3. Wind direction should be within 30 degrees normal to the range."
(2)
Actually several waves may form with some many miles downwind from the mountain causing them. The rising portion of these standing waves (the wave itself is stationary although the air is moving through it at high speed) often provides a spectacular source of lift. Air rising in a wave obeys the same physical laws as that in a thermal, cooling at 3 C per 1,000 feet. Thus the wave may be marked by a lens shaped cloud, standing lenticular altocumulus, or "lennie", which forms on the upwind side by condensation of moisture as the rising air reaches its dew point and dissipates on the downwind side as the sinking air warms. Directly beneath the wave will be an area of extreme turbulence called the rotor, which may or may not be marked by a tumbling cloud.
Because wave soaring occurs in relatively strong winds it may be possible for the glider to remain in one spot over the ground by heading into the wind. Knowledge of the general configuration of waves will help the pilot explore the wave for best lift and avoid the strong sink in the descending portion of the wave and the turbulence in the rotor. The high altitudes attainable in waves require that the pilot manage physiological factors (e.g. oxygen and temperature) and be alert for adverse weather changes that could affect return to the airport.
Textbook illustrations of mountain wave suggest that the same obstruction could produce simultaneously ridge lift on its upwind side and wave lift downwind. It is also possible for a second obstruction, located appropriately near the wave to augment the wave produced by the upwind obstruction. A pilot flying in such lift might wonder if it was wave lift from the upwind obstruction or ridge lift from the one below. It is also possible for a breeze that is too weak to produce ridge lift to trigger a line of thermals (thermal street) along the ridge. Properly identifying such lift sources is more an exercise in semantics than meteorology, but a basic understanding of the three fundamental lift sources is likely to be beneficial for the pilot in the air and is surely beneficial to the applicant taking a practical test. At the very least, applicants should know that ridge lift is on the upwind side of the obstruction, wave lift is on the downwind side (that's why textbooks call them "lee waves"), and thermals can occur anywhere.
An understanding of weather and how to obtain weather information is of little value unless the pilot can apply that knowledge to flight planning. All pilots should recognize and avoid the risks associated with severe weather such as thunderstorms, but the glider pilot's view of some weather conditions may be different from that of airplane pilots. Weather conditions conducive to wave flight might be cause to cancel a cross-country airplane flight in the area, and a large negative thermal index might suggest more turbulence than an airplane pilot wants to endure. Timing of the use of lift sources may be critical to the success of a glider cross-country flight; if a strong headwind must be encountered during a portion of the flight it would probably be best if that leg could be flown when thermals are strongest, saving weak lift for easy downwind legs.
© 2005 Jim D. Burch 602-942-2734 jdburch@att.net
To return to table of contents select TOC